This topic directly to beg to seek out, both in the cartesian coordinates and polar coordinates, because of the integral area is actually a elliptical almost below the shape at an Angle,
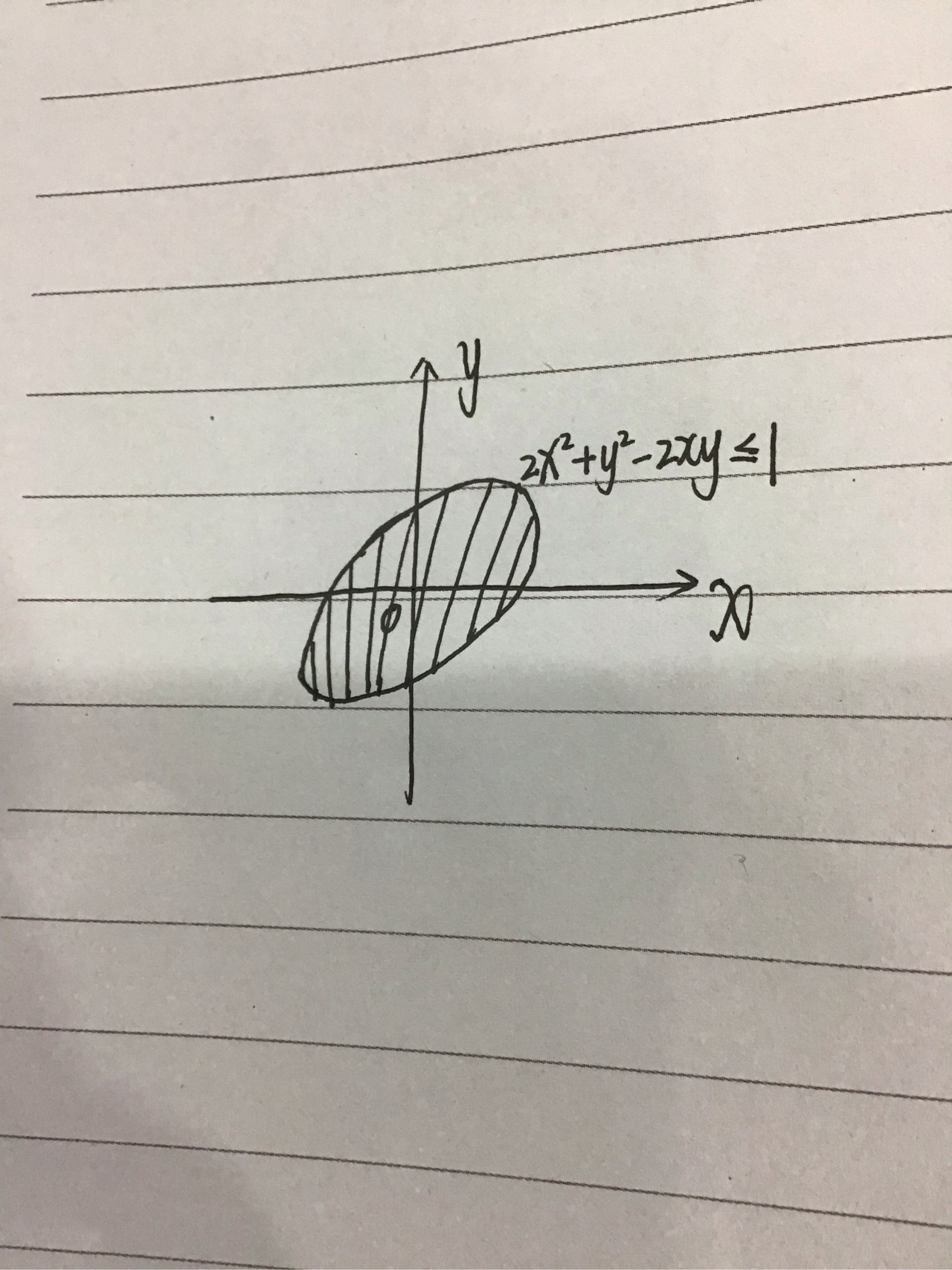
So we'll think of some way to convert the integral area, the original integral area is actually the x2 + (x-y) 2 1 or less, then you can use other variables to replace x and y (x, y), we will use u, v to replace, x=u get equations, x - y=v, then x and y can u and v to say, x=u, y=u - v, the original integral can be written as ∫ ∫ | u |? D | J2 | du dv, of which the integral area for {(u, v) | u2 + v2} 1 or less, then remove the absolute value symbol, on the original integral block points. But I don't know that right, I get is the result of the four thirds. The

CodePudding user response:

For the (round up six words)