how to apply a affine transformation to a single dot in PIL?-CodePudding

In PIL I have rotated a rectangle by an angle and pasted it back to the background image. I'd like to know what will be the new coordinates of a specific corner.

function(old_coord) => new coordinates

I have read in the documentation it mentioned the center of rotation by default is the center of the image.

Here is my code:

import PIL
from PIL import Image, ImageDraw
import os

background = Image.open('background.jpg')
rectangle = Image.open("rectangle.png")
my_angle = 30

rectangle_rotate = Image.Image.rotate(rectangle, angle=my_angle, resample=Image.BICUBIC, expand=True)

# box: 2-tuple giving the upper left corner
px = int(background.size[0] / 2)
py = int(background.size[1] / 2)
background.paste(im=rectangle_rotate,
                     box=(px, py),
                     mask=rectangle_rotate)
# The new position I'm getting is wrong, how come?????
pos_xNew = px * math.cos(math.radians(my_angle))   py * math.sin(math.radians(my_angle))
pos_yNew = -px * math.sin(math.radians(my_angle))   py * math.cos(math.radians(my_angle))
print('pos_xNew:', pos_xNew)
print('pos_yNew:', pos_yNew)
draw_img_pts = ImageDraw.Draw(background)
r = 10
# Drawing a simple small circle circle for visualization
draw_img_pts.ellipse((pos_xNew - r, pos_yNew - r, pos_xNew   r, pos_yNew   r), fill='red')

background.save('example_with_roatation.png')

how can I find the new coordinates value? I keep getting wrong value.

Background image (input):

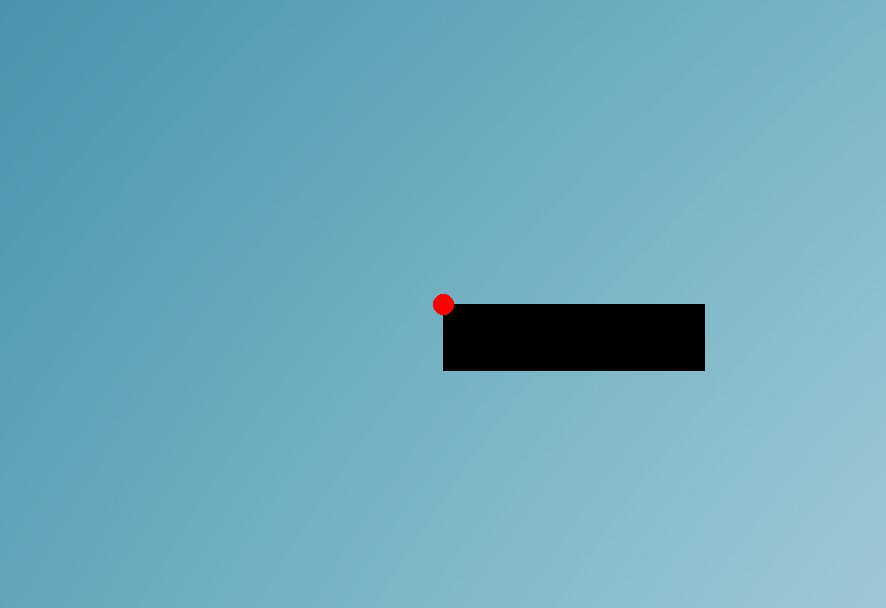
Rectangle image (input):

The Output I got with zero rotation as expected:

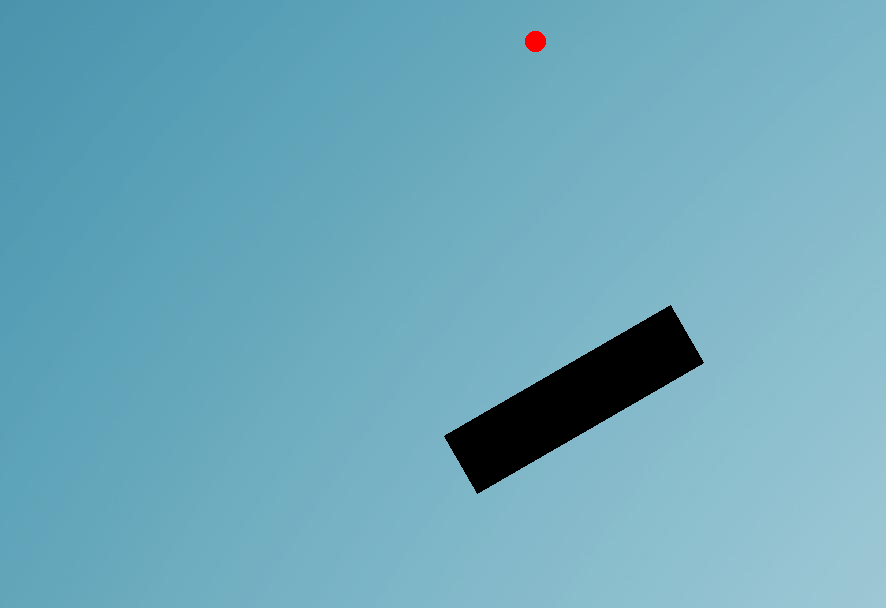
Output I got after 30 degree rotation:

CodePudding user response：

Comments inline

import math
import os

import numpy as np  # many operations are more concise in matrix form
import PIL
from PIL import Image, ImageDraw

def get_rotation_matrix(angle):
    """ For background, https://en.wikipedia.org/wiki/Rotation_matrix
    rotation is clockwise in traditional descartes, and counterclockwise, 
    if y goes down (as in picture coordinates)
    """
    return np.array([
        [np.cos(angle), -np.sin(angle)],
        [np.sin(angle), np.cos(angle)]])

def rotate(points, pivot, angle):
    """ Get coordinates of points rotated by a given angle counterclocwise 
    
    Args:
        points (np.array): point coordinates shaped (n, 2)
        pivot (np.array): [x, y] coordinates of rotation center
        angle (float): counterclockwise rotation angle in radians
    Returns:
        np.array of new coordinates shaped (n, 2)
    """
    relative_points = points - pivot
    return relative_points.dot(get_rotation_matrix(angle))   pivot

background = Image.open('background.jpg')
rectangle = Image.open("rectangle.png")
my_angle_deg = 30
my_angle = math.radians(my_angle_deg)

rsize_x, rsize_y = rectangle.size
# to get shift introduced by rotation clipping we'll need to rotate all four corners
# starting from top-right corners, counter-clockwise
rectangle_corners = np.array([
    [rsize_x, 0],  # top-right
    [0, 0],  # top-left
    [0, rsize_y],  # bottom-left
    [rsize_x, rsize_y]  # bottom-right
])
# rectangle_corners now are:
# array([[262,   0],
#       [  0,   0],
#       [  0,  67],
#       [262,  67]])

rotated_corners = rotate(rectangle_corners, rectangle_corners[0], my_angle)
# as a result of rotation, one of the corners might end up left from 0,
# e.g. if the rectangle is really tall and rotated 90 degrees right
# or, leftmost corner is no longer at 0, so part of the canvas is clipped
shift_introduced_by_rotation_clip = rotated_corners.min(axis=0)

rotated_shifted_corners = rotated_corners - shift_introduced_by_rotation_clip

# finally, demo
# this part is just copied from the question
rectangle_rotate = Image.Image.rotate(rectangle, angle=my_angle_deg, resample=Image.BICUBIC, expand=True)

# box: 2-tuple giving the upper left corner
px = int(background.size[0] / 2)
py = int(background.size[1] / 2)
background.paste(im=rectangle_rotate,
                     box=(px, py),
                     mask=rectangle_rotate)

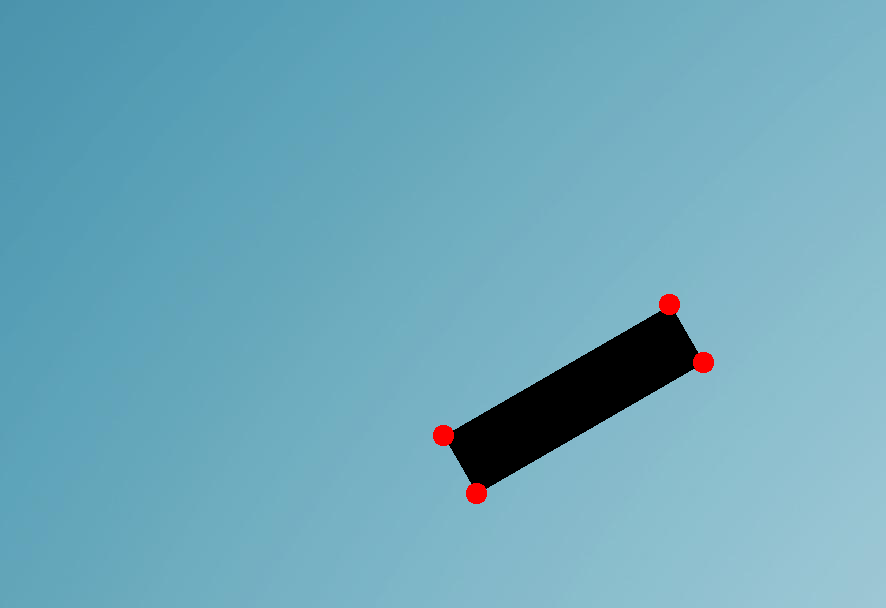
# let's see if dots land right after these translations:
draw_img_pts = ImageDraw.Draw(background)
r = 10
for point in rotated_shifted_corners:
    pos_xNew, pos_yNew = point   [px, py]
    draw_img_pts.ellipse((pos_xNew - r, pos_yNew - r, pos_xNew   r, pos_yNew   r), fill='red')