I want to create an arc trajectory cross over n=3 points P(n)=(x, y, z), I decided to draw a circle over 3 points in plane. so I have center, radius, theta (angle in x, y plane) and phi(angle around z axis), and I know the position of 3 points (x, y, z), How can I extract an arc between p1 , p2 and p3 from this circle? I implemented this program in MATLAB.. Thanks a lot.

CodePudding user response：

This answer on math.stackexchange gives a nice simple formulation for finding the circle centre (and therefore the radius)

% Original points
p1 = [1;0;2];
p2 = [0;0;0];
p3 = [1;2;2];
P = [p1,p2,p3];

% Get circle definition and 3D planar normal to it
p0 = getCentre(p1,p2,p3);
r = norm( p0 - p1 );
[n0,idx] = getNormal(p0,p1,p2,p3);
% Vectors to describe the plane
q1 = P(:,idx(1));
q2 = p0   cross(n0,(p1-p0).').';
% Function to compute circle point at given angle
fc = @(a) p0   cos(a).*(q1-p0)   sin(a).*(q2-p0);
% Get angles of the original points for the circle formula
a1 = angleFromPoint(p0,p1,q1,q2);
a2 = angleFromPoint(p0,p2,q1,q2);
a3 = angleFromPoint(p0,p3,q1,q2);
% Plot
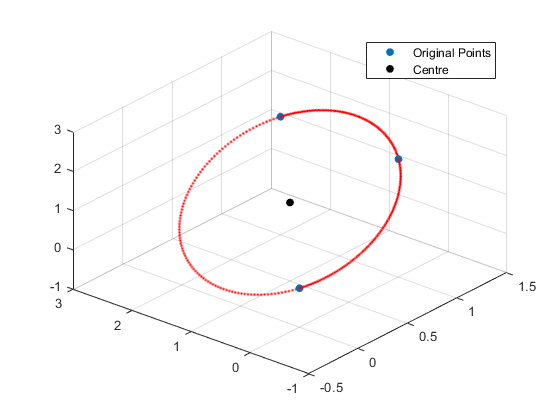
figure(1); clf; hold on;
args = {'markersize',20,'displayname'};
plot3( P(1,:), P(2,:), P(3,:), '.', args{:}, 'Original Points' ); 
plot3( p0(1), p0(2), p0(3), '.k', args{:}, 'Centre' );   
plotArc(fc,a1,a2); % plot arc from p1 to p2
plotArc(fc,a2,a3); % plot arc from p2 to p3
plotArc(fc,a3,a1); % plot arc from p3 to p1
grid on; legend show; view(-50,40);

function ang = angleFromPoint(p0,p,q1,q2)
    % Get the circle angle for point 'p'
    comp = @(a,b) dot(a,b)/norm(b);
    ang = atan2( comp(p-p0,q2-p0), comp(p-p0,q1-p0) );
end
function plotArc(fc,a,b)
    % Plot circle arc between angles 'a' and 'b' for circle function 'fc'
    while a > b
        a = a - 2*pi; % ensure we always go from a to b
    end
    aa = linspace( a, b, 100 );
    c = fc(aa);
    plot3( c(1,:), c(2,:), c(3,:), '.r', 'markersize', 5, 'handlevisibility', 'off' );
end
function p0 = getCentre(p1,p2,p3)
    % Get centre of circle defined by 3D points 'p1','p2','p3'
    v1 = p2 - p1;
    v2 = p3 - p1;

    v11 = dot( v1.', v1 );
    v22 = dot( v2.', v2 );
    v12 = dot( v1.', v2 );

    b = 1/(2*(v11*v22-v12^2));
    k1 = b * v22 * (v11-v12);
    k2 = b * v11 * (v22-v12);

    p0 = p1   k1*v1   k2*v2;
end
function [n0,idx] = getNormal(p0,p1,p2,p3)
    % compute all 3 normals in case two points are colinear with centre
    n12 = cross((p1 - p0),(p2 - p0));
    n23 = cross((p3 - p0),(p2 - p0));
    n13 = cross((p3 - p0),(p1 - p0));

    n = [n12,n23,n13];
    n = n./sign(n(1,:));
    idx = find(~all(isnan(n)),2);
    n = n(:,idx(1));
    n0 = n / norm(n);
end