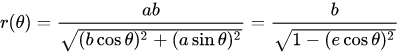the following code is creating svg paths to split a circle into 8 or 24 sections with the same angle at the center of the circle. The factor is only used to create paths that end at the border of the outer rectangle so we end up having a rectangle around the end of all paths.

function get8Or24PiePath(is24Pie, pieChartKind, width, height, customAngle) {
    let resultPath = '';
    const maxIndex = is24Pie ? 12 : 4;
    const circleAngleStep = (2 * Math.PI) / (maxIndex * 2);
    const additionalIndexValue = (customAngle / 360) * (maxIndex * 2);

    for (let index = 0; index < maxIndex; index  ) {
        if (!is24Pie || (index   2) % 3 !== 0) {
            const fromIndex = index   0.5   additionalIndexValue;
            const toIndex = fromIndex   maxIndex;
            const factorFrom =
                pieChartKind === 'S' || pieChartKind === 'R'
                    ? Math.sqrt(1.0) /
                      Math.max(
                            Math.abs(Math.cos(circleAngleStep * fromIndex)),
                            Math.abs(Math.sin(circleAngleStep * fromIndex)),
                      )
                    : 1;
            const factorTo =
                pieChartKind === 'S' || pieChartKind === 'R'
                    ? Math.sqrt(1.0) /
                      Math.max(
                            Math.abs(Math.cos(circleAngleStep * toIndex)),
                            Math.abs(Math.sin(circleAngleStep * toIndex)),
                      )
                    : 1;
            const xFrom =
                factorFrom * Math.cos(circleAngleStep * fromIndex) * (width * 0.5)   width * 0.5;
            const yFrom =
                factorFrom * Math.sin(circleAngleStep * fromIndex) * (height * 0.5)   height * 0.5;
            const xTo =
                factorTo * Math.cos(circleAngleStep * toIndex) * (width * 0.5)   width * 0.5;
            const yTo =
                factorTo * Math.sin(circleAngleStep * toIndex) * (height * 0.5)   height * 0.5;

            resultPath  = `M ${xFrom} ${yFrom} L ${xTo} ${yTo} `;
        }
    }

    return resultPath;
}

ro(theta) = a * b / sqrt(a^2*sin^2(theta)   b^2*cos^2(theta))   
x(theta) = ro * cos(theta)
y(theta) = ro * sin(theta)