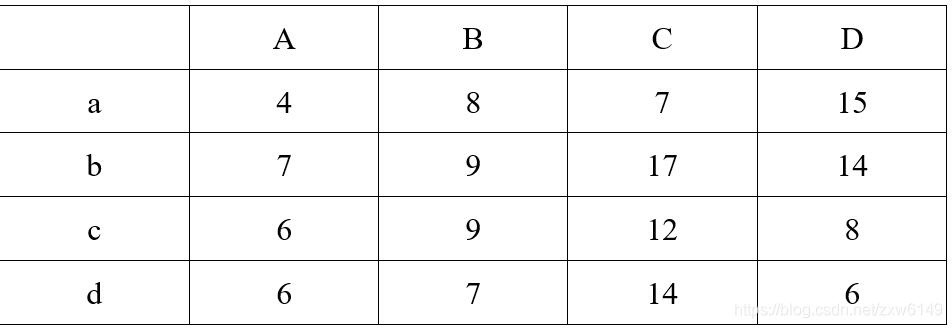
Ideally (without obstacles, regardless of the collision), four same type cars (a, b, c, d) from any place and at the same time, respectively to reach 4 specifies the location of the garage (a, b, c, d) (a garage can stop a car), assuming that all the time the car into the library is T, namely complete storage time, the last car programming to find the minimum value of T, and an optimal scheme is given, the corresponding relationship and the garage, the car storage time shown in the table below, here want to use a greedy algorithm, don't know why a bit of a problem

#include
using namespace std; Int main ()
{int a [4] [4]. Int I, j, x, y, t, tx=1, t=0; for(j=0; J<4. {j++) for (I=0; i<4. I++) {cin> A, [I] [j]. }} cout<& lt;" The end!" }